Alignment and Balancing
For most rotating machines used in the process industries, the trend is toward higher speeds, higher horsepowers per machine, and less sparing. The first of these factors increases the need for precise balancing and alignment. This is necessary to minimize vibration and premature wear of bearings, couplings, and shaft seals. The latter two factors increase the economic importance of high machine reliability, which is directly dependent on minimizing premature wear and breakdown of key components.
Balancing, deservedly, has long received attention from machinery manufacturers and users as a way to minimize vibration and wear. Many shop and field balancing machines, instruments, and methods have become available over the years. Alignment, which is equally important, has received proportionately less notice than its importance justifies. Any kind of alignment, even straightedge alignment, is better than no alignment at all. Precise, two-indicator alignment is better than rough alignment, particularly for machines 3600 RPM and higher. It can give greatly improved bearing and seal life, lower vibration, and better overall reliability. It does take longer, however, especially the first time it is done to a particular machine, or when done by inexperienced personnel. The process operators and mechanical supervisors must be made aware of this time requirement. If they insist on having the job done in a hurry. they should do so with full knowledge of the likelihood of poor alignment and reduced machine reliability. Figure 5-1 shows a serious machinery failure which started with piping-induced misalignment, progressed to coupling distress, bearing failure, and finally, total wreck.
Balancing, deservedly, has long received attention from machinery manufacturers and users as a way to minimize vibration and wear. Many shop and field balancing machines, instruments, and methods have become available over the years. Alignment, which is equally important, has received proportionately less notice than its importance justifies. Any kind of alignment, even straightedge alignment, is better than no alignment at all. Precise, two-indicator alignment is better than rough alignment, particularly for machines 3600 RPM and higher. It can give greatly improved bearing and seal life, lower vibration, and better overall reliability. It does take longer, however, especially the first time it is done to a particular machine, or when done by inexperienced personnel. The process operators and mechanical supervisors must be made aware of this time requirement. If they insist on having the job done in a hurry. they should do so with full knowledge of the likelihood of poor alignment and reduced machine reliability. Figure 5-1 shows a serious machinery failure which started with piping-induced misalignment, progressed to coupling distress, bearing failure, and finally, total wreck.
Prealignment Requirements
The most important requirement is to have someone who knows what he is doing, and cares enough to do it right. Continuity is another important factor. Even with good people, frequent movement from location to location can cause neglect of things such as tooling completeness and prealignment requirements.
The saying that “you can’t make a silk purse out of a sow’s ear” also applies to machinery alignment. Before undertaking an alignment job, it is prudent to check for other deficiencies which would largely nullify the benefits or prevent the attainment and retention of good alignment. Here is a list of such items and questions to ask oneself: Foundation Adequate size and good condition? A rule of thumb calls for concrete weight equal to three times machine weight for rotating machines, and five times for reciprocating machines. Grout Suitable material, good condition, with no voids remaining beneath baseplate? Tapping with a small hammer can detect hollow spots, which can then be filled by epoxy injection or other means. This is a lot of trouble, though, and often is not necessary if the lack of grout is not causing vibration or alignment drift.
Baseplate Designed for adequate rigidity? Machine mounting pads level, flat, parallel, coplanar, clean? Check with straightedge and feeler gauge. Do this upon receipt of new pumps, to make shop correction possible-and maybe collect the cost from the pump manufacturer. Shims clean, of adequate thickness, and of corrosion- and crush-resistant material? If commercial pre-cut shims are used, check for actual versus marked thicknesses to avoid a soft foot condition. Machine hold-down bolts of adequate size, with clearance to permit alignment corrective movement‘? Pad height leaving at least 2 in. jacking clearance beneath center at each end of machine element to be adjusted for alignment? If jackscrews are required, are they mounted with legs sufficiently rigid to avoid deflection? Are they made of type 3 16 stainless steel, or other suitable material, to resist field corrosion? Water or oil cooled or heated pedestals are usually unnecessary, but can in some cases be used for onstream alignment thermal compensation.
Piping Is connecting piping well fitted and supported, and sufficiently flexible, so that no more than 0.003 in. vertical and horizontal (measured separately -not total) movement occurs at the flexible coupling when the last pipe flanges are tightened? Selective flange bolt tightening may be required, while watching indicators at the coupling. If pipe flange angular misalignment exists, a “dutchman” or tapered filler piece may be necessary. To determine filler piece dimensions,

measure flange gap around circumference, then calculate as follows:
Gasket 0. D . I Flange O.D. I = l/8 in. + (Max. Gap - Min. Gap) Maximum Thickness of Tapered Filler Piece1/8 in. = Dutchman Minimum Thickness (180’ from Maximum Thickness). Dutchman OD and ID same as gasket OD and ID.
Spiral wound gaskets may be helpful, in addition to or instead of a tapered filler piece. Excessive parallel offset at the machine flange connection cannot be cured with a filler piece. It may be possible to absorb it by offsetting several successive joints slightly, taking advantage of clearance between flange bolts and their holes. If excessive offset remains, the piping should be bent to achieve better fit. For the “stationary” machine
element, the piping may be connected either before or after the alignment is done-provided the foregoing precautions are taken, and final alignment remains within acceptable tolerances. In some cases, pipe expansion or movement may cause machine movement leading to misalignment and increased vibration. Better pipe supports or stabilizers may be needed in such situations. At times it may be necessary to adjust these components with the machine running, thus aligning the machine to get minimum vibration. Sometimes, changing to a more tolerant type of coupling, such as elastomeric, may help.
Coupling Some authorities recommend installation on typical pumps Installation and drivers with an interference fit, up to .OW5 in. per in. of shaft diameter. In our experience, this can give problems in subsequent removal or axial adjustment. If an interference fit is to be used, we prefer a light one-say .OOO3 in. to .OOO5 in. overall, regardless of diameter. For the majority of machines operating at 3600 RPM and below, you can install couplings with .0005 in. overall diametral clearance, using a setscrew over the keyway. For hydraulic dilation couplings and other nonpump or special categories, see manufacturers’ recommendations or appropriate section of this text. Many times, high-performance couplings require interference fits as high as .0025 in. per in. of shaft diameter.
Coupling cleanliness, and for some types, lubrication, are important and should be considered. Sending a repaired machine to the field with its lubricated coupling-half unprotected, invites lubricant contamination, rusting, dirt accumulation, and premature failure. Lubricant should be chosen from among those recommended by the coupling manufacturer or a reputable oil company. Continuous running beyond two years is inadvisable without inspecting a grease lubricated coupling, since the centrifuging effects are likely to cause caking and loss of lubricity. Certain lubricants, e.g., Amoco and Koppers coupling greases, are reported to eliminate this problem, but visual external inspection is still advisable to detect leakage. Continuous lube couplings are subject to similar problems, although such remedies as anti-sludge holes can be used to allow longer runs at higher speeds. By far the best remedy is clean oil, because even small amounts of water will promote sludge formation. Spacer length can be important, since parallel misalignment accommodation is directly proportional to such length.
Coupling Some authorities recommend installation on typical pumps Installation and drivers with an interference fit, up to .OW5 in. per in. of shaft diameter. In our experience, this can give problems in subsequent removal or axial adjustment. If an interference fit is to be used, we prefer a light one-say .OOO3 in. to .OOO5 in. overall, regardless of diameter. For the majority of machines operating at 3600 RPM and below, you can install couplings with .0005 in. overall diametral clearance, using a setscrew over the keyway. For hydraulic dilation couplings and other nonpump or special categories, see manufacturers’ recommendations or appropriate section of this text. Many times, high-performance couplings require interference fits as high as .0025 in. per in. of shaft diameter.
Coupling cleanliness, and for some types, lubrication, are important and should be considered. Sending a repaired machine to the field with its lubricated coupling-half unprotected, invites lubricant contamination, rusting, dirt accumulation, and premature failure. Lubricant should be chosen from among those recommended by the coupling manufacturer or a reputable oil company. Continuous running beyond two years is inadvisable without inspecting a grease lubricated coupling, since the centrifuging effects are likely to cause caking and loss of lubricity. Certain lubricants, e.g., Amoco and Koppers coupling greases, are reported to eliminate this problem, but visual external inspection is still advisable to detect leakage. Continuous lube couplings are subject to similar problems, although such remedies as anti-sludge holes can be used to allow longer runs at higher speeds. By far the best remedy is clean oil, because even small amounts of water will promote sludge formation. Spacer length can be important, since parallel misalignment accommodation is directly proportional to such length.
Alignment Tolerances
Before doing an alignment job, we must have tolerances to work toward. Otherwise, we will not know when to stop. One type of “tolerance” makes time the determining factor, especially on a machine that is critical to plant operation, perhaps the only one of its kind. The operations superintendent may only be interested in getting the machine back on the line,fast. If his influence is sufficient, the job may be hurried and done to rather loose alignment tolerances. This can be unfortunate, since it may cause excessive vibration, premature wear, and early failure. This gets us back to the need for having the tools and knowledge for doing a good alignment job efficiently. So much for the propaganda-now for the tolerances.
Tolerances must be established before alignment, in order to know when to stop. Various tolerance bases exist. One authority recommends ‘h-mil maximum centerline offset per in. of coupling length, for hot running misalignment. A number of manufacturers have graphs which recommend tolerances based on coupling span and speed. A common tolerance in terms of face-and-rim measurements is .003-in. allowable face gap difference and centerline offset. This ignores the resulting accuracy variation due to face diameter and spacer length differences, but works adequately for many machines.
Be cautious in using alignment tolerances given by coupling manufacturers. These are sometimes rather liberal and, while perhaps true for the coupling itself, may be excessive for the coupled machinery. A better guideline is illustrated in Figure 5-2, which shows an upper, absolute misalignment limit, and a lower, “don’t exceed for good longterm operation limit.” The real criterion is the running vibration. If excessive, particularly at twice running frequency and axially, further alignment improvement is probably required. Analysis of failed components such as bearings, couplings, and seals can also indicate the need for improved alignment.
Before doing an alignment job, we must have tolerances to work toward. Otherwise, we will not know when to stop. One type of “tolerance” makes time the determining factor, especially on a machine that is critical to plant operation, perhaps the only one of its kind. The operations superintendent may only be interested in getting the machine back on the line,fast. If his influence is sufficient, the job may be hurried and done to rather loose alignment tolerances. This can be unfortunate, since it may cause excessive vibration, premature wear, and early failure. This gets us back to the need for having the tools and knowledge for doing a good alignment job efficiently. So much for the propaganda-now for the tolerances.
Tolerances must be established before alignment, in order to know when to stop. Various tolerance bases exist. One authority recommends ‘h-mil maximum centerline offset per in. of coupling length, for hot running misalignment. A number of manufacturers have graphs which recommend tolerances based on coupling span and speed. A common tolerance in terms of face-and-rim measurements is .003-in. allowable face gap difference and centerline offset. This ignores the resulting accuracy variation due to face diameter and spacer length differences, but works adequately for many machines.
Be cautious in using alignment tolerances given by coupling manufacturers. These are sometimes rather liberal and, while perhaps true for the coupling itself, may be excessive for the coupled machinery. A better guideline is illustrated in Figure 5-2, which shows an upper, absolute misalignment limit, and a lower, “don’t exceed for good longterm operation limit.” The real criterion is the running vibration. If excessive, particularly at twice running frequency and axially, further alignment improvement is probably required. Analysis of failed components such as bearings, couplings, and seals can also indicate the need for improved alignment.

Figure 5-2 can be applied to determine allowable misalignment for machinery equipped with nonlubricated metal disc and diaphragm couplings, up to perhaps 10,000 rpm. If the machinery is furnished with gear-type couplings, Figure 5-2 should be used up to 3,600 rpm only. At speeds higher than 3,600 rpm, gear couplings will tolerate with impunity only those shaft misalignments which limit the sliding velocity of engaging gear teeth to less than perhaps 120 in. per minute. For gear couplings, this velocity can be approximated by V = (TDN) tancr, where
resimmmmmm Say, for example, we were dealing with a 3560 rpm pump coupled to a motor driven via a 6-in. pitch diameter gear coupling. We observe a total indicator reading of 26 mils in the vertical plane and a total indicator reading of 12 mils in the horizontal plane. The distance between the flexing member of the coupling, i.e., flexing member on driver and flexing member on driven machine, is 10 in. The total net indicator reading is [(26)2 + (12)?]''* = 28.6 mils. Tan CY = (1/2)(28.6)/10) = 1.43 milslin., or 0.00143 in./in. The sliding velocity is therefore [(~)(6)(3560) (0.00143)1 = 96 in. per minute. Since this is below the maximum allowable sliding velocity of 120 in. per minute, the installation would be within allowable misalignment.
Choosing an Alignment Measurement Setup
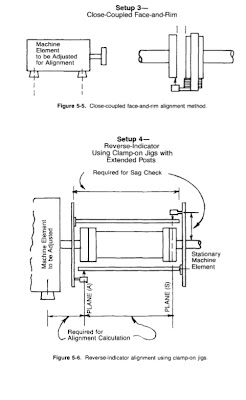
Having taken care of the preliminaries, we are now ready to choose an alignment setup, or arrangement of measuring instruments. Many such setups are possible, generally falling into three broad categories: faceand- rim, reverse-indicator, and face-face-distance. The following sketches show several of the more common setups, numbered arbitrarily for ease of future reference. Note that if measurements are taken with calipers or ID micrometers, it may be necessary to reverse the sign from that which would apply if dial indicators are used. Figures 5-3 through 5-8 show several common arrangements of indicators, jigs, etc. Other arrangements are also possible. For example, Figures 5-3 and 5-4 can be done with jigs, either with or without breaking the coupling. They can also sometimes be done when no spacer is present, by using right-angle indicator extension tips. Figures 5-6 and 5-7 can be set up with both extension arms and indicators on the same side,
rather than 180" opposite as shown. In such cases, however, a sign reversal will occur in the calculations. Also, we can indicate on back of face, as for connected metal disc couplings. Again, a sign reversal will occur. In choosing the setup to use, personal preference and custom will naturally influence the decision, but here are some basic guidelines to follow.
Reverse-Indicator Method
This is the setup we prefer for most alignment work. As illustrated in Figure 5-9, it has several advantages:
1. Accuracy is not affected by axial movement of shafts in sleeve bearings.
2. Both shafts turn together, either coupled or with match marks, so coupling eccentricity and surface irregularities do not reduce accuracy of alignment readings.
3. Face alignment, if desired, can be derived quite easily without direct measurement.
Reverse-Indicator Method
This is the setup we prefer for most alignment work. As illustrated in Figure 5-9, it has several advantages:
1. Accuracy is not affected by axial movement of shafts in sleeve bearings.
2. Both shafts turn together, either coupled or with match marks, so coupling eccentricity and surface irregularities do not reduce accuracy of alignment readings.
3. Face alignment, if desired, can be derived quite easily without direct measurement.
4. Rim measurements are easy to calibrate for bracket sag. Face sag, by contrast, is considerably more complex to measure.
5. Geometric accuracy is usually better with reverse-indicator method in process plants, where most couplings have spacers.
6. With suitable clamp-on jigs, the reverse-indicator method can be used quite easily for measuring without disconnecting the coupling or removing its spacer. This saves time, and for gear couplings, reduces the chance for lubricant contamination.
7. For the more complex alignment situations, where thermal growth and/or multi-element trains are involved, reverse-indicator can be used quite readily to draw graphical plots showing alignment conditions and moves. It is also useful for calculating optimum moves of two or more machine elements, when physical limits do not allow full correction to be made by moving a single element.
8. When used with jigs and posts, single-axis leveling is sufficient for ball-bearing machines, and two-axis leveling will suffice for sleeve-bearing machines.
9. For long spans, adjustable clamp-on jigs are available for reverseindicator application, without requiring coupling spacer removal. Face-and-rim jigs for long spans, by contrast, are usually nonadjustable custom brackets requiring spacer removal to permit face mounting.
10. With the reverse-indicator setup, we mount only one indicator per bracket, thus reducing sag as compared to face-and-rim, which mounts two indicators per bracket. (Face-and-rim can do it with one per bracket if we use two brackets, or if we remount indicators and rotate a second time, but this is more trouble.)
There are some limitations of the reverse-indicator method. It should not be used on close-coupled installations, unless jigs can be attached behind the couplings to extend the span to 3 in. or more. Failure to observe this limitation will usually result in calculated moves which overcorrect for the misalignment.
Both coupled shafts must be rotatable, preferably by hand, and preferably while coupled together. If only one shaft can be rotated, or if neither can be rotated, the reverse-indicator method cannot be used. If the coupling diameter exceeds available axial measurement span, geometric accuracy will be poorer with reverse-indicator than with faceand- rim.
If required span exceeds jig span capability, either get a bigger jig or change to a different measurement setup such as face-face-distance. Cooling tower drives would be an example of this.
Face-and-Rim Method
This is the “traditional” setup which is probably the most popular, al- Advantages of face-and-rim:
1. It can be used on large, heavy machines whose shafts cannot be
2. It has better geometric accuracy than reverse-indicator, for large di-
3. It is easier to apply on short-span and small machines than is rethough it is losing favor as more people learn about reverse-indicator. turned. ameter couplings with short spans.
verse-indicator, and will often give better accuracy. Limitations of face-and-rim: 1. If used on a machine in which one or both shafts cannot be turned, some runout error may occur, due to shaft or coupling eccentricity.
If used on a sleeve bearing machine, axial float error may occur. One method of avoiding this is to bump the turned shaft against the axial stop each time before reading. Another way is to use a second face indicator 180" around from the first, and take half the algebraic difference of the two face readings after 180" rotation from zero start. Figure 5-10 illustrates this alignment method. Two 2411. tubular graphite jigs are used for light weight and high rigidity. If used with jigs and posts, two or three axis leveling is required, for ball and sleeve bearing machines respectively. Reverse-indicator requires leveling in one less axis for each.
Face-and-rim has lower geometric accuracy than reverse-indicator, for spans exceeding coupling or jig diameter.
Face sag is often insignificant, but it can occur on some setups, and result in errors if not accounted for. Calibration for face sag is considerably more complex than for rim sag.
For long spans, face-and-rim jigs are usually custom-built brackets requiring spacer removal to permit face mounting. Long-span reverse- indicator jigs, by contrast, are available in adjustable clampon models not requiring spacer removal.
Graphing the results of face-and-rim measurements is more complex than with reverse-indicator measurements.
5. Geometric accuracy is usually better with reverse-indicator method in process plants, where most couplings have spacers.
6. With suitable clamp-on jigs, the reverse-indicator method can be used quite easily for measuring without disconnecting the coupling or removing its spacer. This saves time, and for gear couplings, reduces the chance for lubricant contamination.
7. For the more complex alignment situations, where thermal growth and/or multi-element trains are involved, reverse-indicator can be used quite readily to draw graphical plots showing alignment conditions and moves. It is also useful for calculating optimum moves of two or more machine elements, when physical limits do not allow full correction to be made by moving a single element.
8. When used with jigs and posts, single-axis leveling is sufficient for ball-bearing machines, and two-axis leveling will suffice for sleeve-bearing machines.
9. For long spans, adjustable clamp-on jigs are available for reverseindicator application, without requiring coupling spacer removal. Face-and-rim jigs for long spans, by contrast, are usually nonadjustable custom brackets requiring spacer removal to permit face mounting.
10. With the reverse-indicator setup, we mount only one indicator per bracket, thus reducing sag as compared to face-and-rim, which mounts two indicators per bracket. (Face-and-rim can do it with one per bracket if we use two brackets, or if we remount indicators and rotate a second time, but this is more trouble.)
There are some limitations of the reverse-indicator method. It should not be used on close-coupled installations, unless jigs can be attached behind the couplings to extend the span to 3 in. or more. Failure to observe this limitation will usually result in calculated moves which overcorrect for the misalignment.
Both coupled shafts must be rotatable, preferably by hand, and preferably while coupled together. If only one shaft can be rotated, or if neither can be rotated, the reverse-indicator method cannot be used. If the coupling diameter exceeds available axial measurement span, geometric accuracy will be poorer with reverse-indicator than with faceand- rim.
If required span exceeds jig span capability, either get a bigger jig or change to a different measurement setup such as face-face-distance. Cooling tower drives would be an example of this.
Face-and-Rim Method
This is the “traditional” setup which is probably the most popular, al- Advantages of face-and-rim:
1. It can be used on large, heavy machines whose shafts cannot be
2. It has better geometric accuracy than reverse-indicator, for large di-
3. It is easier to apply on short-span and small machines than is rethough it is losing favor as more people learn about reverse-indicator. turned. ameter couplings with short spans.
verse-indicator, and will often give better accuracy. Limitations of face-and-rim: 1. If used on a machine in which one or both shafts cannot be turned, some runout error may occur, due to shaft or coupling eccentricity.
If used on a sleeve bearing machine, axial float error may occur. One method of avoiding this is to bump the turned shaft against the axial stop each time before reading. Another way is to use a second face indicator 180" around from the first, and take half the algebraic difference of the two face readings after 180" rotation from zero start. Figure 5-10 illustrates this alignment method. Two 2411. tubular graphite jigs are used for light weight and high rigidity. If used with jigs and posts, two or three axis leveling is required, for ball and sleeve bearing machines respectively. Reverse-indicator requires leveling in one less axis for each.
Face-and-rim has lower geometric accuracy than reverse-indicator, for spans exceeding coupling or jig diameter.
Face sag is often insignificant, but it can occur on some setups, and result in errors if not accounted for. Calibration for face sag is considerably more complex than for rim sag.
For long spans, face-and-rim jigs are usually custom-built brackets requiring spacer removal to permit face mounting. Long-span reverse- indicator jigs, by contrast, are available in adjustable clampon models not requiring spacer removal.
Graphing the results of face-and-rim measurements is more complex than with reverse-indicator measurements.
Face-Face-Distance Method
Advantages of face-face-distance:
1. It is usable on long spans, such as cooling tower drives, without elaborate long-span brackets or consideration of bracket sag.
2. It is the basis for thermal growth measurement in the Indikon proximity probe system, and again is unaffected by long axial spans.
3. It is sometimes a convenient method for use with diaphragm couplings such as Bendix and Koppers, allowing mounting of indicator holders on spacer tube, with indicator contact points on diaphragm covers.
Limitations of face-face-distance:
1. It has no advantage over the other methods for anything except long spans.
2. It cannot be used for installations where no coupling spacer is present.
3. Its geometric accuracy will normally be lower than either of the other two methods.
4. It may or may not be affected by axial shaft movement in sleeve bearings, but this can be avoided by the same techniques as for face-and-rim.
Laser-Optic Allgnment
In the early 1980’s, by means of earth-bound laser beams and a reflector mounted on the moon, man has determined the distance between earth and the moon to within about 6 inches. Such accuracy is a feature of optical measurement systems, as light travels through space in straight lines, and a bundled laser ray with particular precision.
Thus, critical machinery alignment, where accuracy of measurement is of paramount importance, is an ideal application for a laser-optic alignment system.
The inherent problems of mechanical procedure and sequence of measuring have been solved by Prueftechnik Dieter Busch, of 8045 Ismaning (West Germany), whose OPTALIGN@ system comprises a semiconductor laser emitting a beam in the infrared range (wavelength 820 mm), along with a beamfinder incorporating an infrared detector. The laser beam is refracted through a prism and is caught by a receiver/detector.
These light-weight, nonbulky devices are mounted on the equipmcnt shafts, and only a cord-connected microcomputer module is external to the beam emission and receiverldetector devices. The prism redirects the beam and allows measurement of parallel offset in one plane and angularity in another, thus simultaneously controlling both. In one 360" rotation of the shafts all four directional alignment corrections are determined.
The receiver is a biaxial analog photoelectric semiconductor position detector, yielding mathematical results to within one micron. Data for computation are entered automatically through a cable direct from the receiver/ detector. The only information still to be entered manually is the relative position, 4 times, at 0, 90, 180 and 270".
With the data automatically obtained from the receiver/detector, the microcomputer instantaneously yields the horizontal and vertical adjustment results for the alignment of the machine to be moved.
Physical contact between measuring points on both shafts is no longer required, as this is now bridged by the laser beam, eliminating the possibilities for error arising from gravitational hardware sag as well as from sticky dial indicators, etc. The system's basic attachment is still carried out with a standard quick-fit bracketing system, or with any other suitable attachment hardware.
If the reader owns an OPTALIGN@ system, he does not have to be concerned with sag. Other readers must continue the checkout process.
Checking for Bracket Sag
Long spans between coupling halves may cause the dial indicator fixture to sag measurably because of the weight of the fixture and the dial indicators. Although sag may be minimized by proper bracing, sag effects should still be considered in vertical alignment, To determine sag, install the dial indicators on the alignment fixture in the same orientation and relative position as in the actual alignment procedure with the fixture resting on a level surface as shown in Figure 5-1 I. With a small sling and scale, lift the indicator end of the fixture so that the fixture is in the horizontal position. Note the reading on the scale. Assume for example that the scale reading was 7.5 Ibs. Next, mount the alignment fixture on the coupling hub with the dial indicator plunger touching the top vertical rim of the opposite coupling hub. Set the dial indicator to zero. Next, locate the sling in the same relative position as before and, while observing the scale, apply an upward force so as to repeat the previous scale reading (assumed 7.5 Ibs in our example). Note the dial indicator reading while holding the upward force.
Let us assume for example that we observe a dial indicator reading of - 0.004 in. Using this specific methodology, sag error applies equally to the top and bottom readings. Therefore, the sag correction to the total indicator reading is double the indicated sag and must be algebraically subtracted from the bottom vertical parallel reading, i.e., - (2) ( - .004) = + .008 correction to bottom reading. This method is a clever one for face-mounted brackets. For clamp-on brackets, however, it would be easier and more common to attach them to a horizontal pipe on sawhorses, and roll top to bottom. Figure 5-12 shows this conventional method which, except for the sag compensator device, is almost universally employed. The sag compensator feature incorporates a weight-beam scale which applies an upward force when the indicator bracket is located at the top of the machine shaft, and an equal, but opposite, force when the indicator bracket and shaft combination is rotated to the down position, 180" removed.
In any event, let us assume that we obtain readings of 0 and + 0.160 in. at the top and bottom vertical parallels respectively. We correct for sag in the following manner:
Bracket Sag Effect on Face Measurements
Bracket sag is generally thought to primarily affect rim readings, with little effect on face readings. Often this is true, but some risk may be incurred by assuming this without a test. Unlike rim sag, face sag effect depends not only on jig or bracket stiffness, but on its geometry. Determining face sag effect is fairly easy. First get rim sag for span to be used (we are referring here to the full indicator deflection due to sag when the setup is rotated from top to bottom). This may be obtained by trial, with rim indicator only, or from a graph of sags compiled for the bracket to be used. Then install a setup with rim indicator only, on Calibration pipe or on actual field machine, and “lay on” the face indicator and accessories, noting additional rim indicator deflection when this is done. Double this additional deflection, and add it to the rim sag found previously, if both the face and rim indicators are to be used simultaneously. If the face and the rim indicators are to be used separately, to reduce sag, use the original rim sag in the normal manner, and use this same original rim sag as shortly to be described in determining face sag effect-in this latter case utilizing a rim indicator installed temporarily with the face indicator for this purpose. If the face indicator is a different type (Le., different weight) from the rim indicator, obtain rim sag using this face indicator on the rim, and use this figure to determine face sag effect.
Now install face and rim setup on the actual machine, and zero the indicators. With indicators at the top, deflect bracket upward an amount equal to the appropriate rim sag, reading on the rim indicator, and note the face indicator reading. The face sag correction with indicators at bottom would be this amount with opposite sign. If zeroing the setup at the bottom, the face sag correction at the top would be this amount with same sign (if originally determined at top, as described).
Face Sag Effect-Examples
Example 1
Face and rim indicators are to be used together as shown in Figure 5-3. Assume you will obtain the following from your sag test: Rim sag with rim indicator only = .004 in. Rim sag with two indicators = .007 in. Mount the setup on the machine in the field, and with indicators at top, deflect the bracket upward .007 in. as measured on the rim indicator. When this is done, the face indicator reads plus .002 in. Face sag correction at the bottom position would therefore be minus .002 in. If you wish to zero at the bottom for alignment, but otherwise have data as noted, the face sag correction at the top would be plus .002 in.
Example 2
Face and rim indicators are to be used separately to reduce sag. Both indicators are the same type and weight. Other basic data are also the same.
Install face indicator and temporary rim indicator on the machine in the field, and place in top position. Zero indicators and deflect upward ,004 in. as measured on rim indicator. Face indicator reads plus .0013 in. Face sag correction at the bottom would therefore be minus .0013 in, If zeroing at the bottom for alignment, but otherwise the same as above, face sag correction at top would be plus .0013 in. Example 3
This will determine sag for “3-Indicator Face-and-Rim Setup” shown in Figure 5-4.
Set up the jig to the same geometry as for field installation but with rim indicator only and roll 180” top to bottom on pipe to get total single indicator rim sag - (Step 1).
Zero rim indicator on top and add or “lay on” face indicator, noting rim indicator deflection that occurs - (Step 2). Double this __ (Step 3).
Add it to original total single indicator rim sag (Step 1). - (Step 4). This figure, preceded by a plus sign, will be the sag correction for the rim indicator readings taken at bottom. With field measurement setup as shown, zero all indicators, and deflect the indicator end of the upper bracket upward an amount equal to the total rim sag (Step 4). Note the face sag effect by reading the face indicator. This amount, with opposite sign, is the face sag correction to apply to the readings taken at the lower position - (Step 5). Now deflect the upper bracket back down from its “total rim sag” deflection an amount equal to Step 3.
The amount of sag remaining on the face indicator, preceded by the same sign, is the sag correction for the single face indicator being read at the top position - (Step 6).
All of the foregoing refers of course to bracket sag. In long machines, we will also have shaft sag. This is mentioned only in passing, since there is no need to do anything about it at this time. Our “point-by-point” alignment will automatically take care of shaft sag. For initial leveling of large turbogenerators, etc., especially if using precision optical equipment, shaft sag must be considered. Manufacturers of such machines know this, and provide their erectors with suitable data for sag compensation. Further discussion of shaft sag is beyond the scope of this text.
Leveling Curved Surfaces
It is common practice to set up the “rim” dial indicators so their contact tips rest directly on the surface of coupling rims or shafts. If gross misalignment is not present, and if coupling and/or shaft diameters are large, which is usually the case, accuracy will often be adequate. If, however, major misalignment exists, and/or the rim or shaft diameters are small, a significant error is likely to be present. It occurs due to the measurement surface curvature, as illustrated in Figures 5-13 and 5-14. This error can usually be recognized by repeated failure of top-plusbottom (T + B) readings to equal side-plus-side (S + S) readings within one or two thousandths of an inch, and by calculated corrections resulting in an improvement which undershoots or overshoots and requires repeated corrections to achieve desired tolerance. A way to minimize this error is to use jigs, posts, and accessories which “square the circle.” Here we attach flat surfaces or posts to the curved surfaces, and level

them at top and bottom dead center. This corrects the error as shown in Figure 5-14.
For this method to be fully effective, rotation should be performed at accurate 90” quadrants, using inclinometer or bubble-vial device. In most cases, however, this error is not enough to bother eliminating- it is easier just to make a few more corrective moves, reducing the error each time.
Jig Posts
The preceding explanation showed a rudimentary auxiliary surface, or “jig post,” used for “squaring the circle.” A more common reason for using jig posts is to permit measurement without removing the spacer on a concealed hub gear coupling. If jig posts are used, it is important that they be used properly. In effect, we must ensure that the surfaces contacted by the indicators meet these criteria:
As already shown, they must be leveled in coordination at top and bottom dead centers, to avoid inclined plane error.
If any axial shaft movement can occur, as with sleeve bearings, the surfaces should also be made parallel to their shafts. This can be done by leveling axially at the top, rotating to the bottom, and rechecking. If bubble is not still level, tilt the surface back toward level for a half correction.
If face readings are to be taken on posts, the post face surfaces should be machined perpendicular to their rim surfaces. In addition to this, and to steps 1 and 2 just described, rotate shafts so posts are horizontal. Using a level, adjust face surfaces so they are vertical. Rotate 180” and recheck with level. If not still vertical, tilt back toward vertical to make a half correction on the bubble. This will accomplish our desired objective of getting the face surface perpendicular to the shaft in all measurement planes.
The foregoing assumes use of tri-axially adjustable jig posts. If such posts are not available, it may be possible to get good results using accurately machined nonadjustable posts. If readings and corrections do not turn out as desired, however, it could pay to make the level checks as described-they might pinpoint the problem and suggest a solution such as using a nonpost measurement setup.
interpretation and Data Recording
Due to sag as well as geometry of the machine installation, it is difficult and deceptive to try second-guessing the adequacy of alignment solely from the “raw” indicator readings. It is necessary to correct for sag, then note the “interpreted” readings, then plot or calculate these to see the overall picture-including equivalent face misalignment if primary readings were reverse-indicator on rims only. Sometimes thermal offsets must be included, which further complicates the overall picture. As a way to systematically consider these factors and arrive at a solution, it is helpful to use prepared data forms and stepwise calculation. Suppose we are using the two-indicator face-rim method shown in Figure 5-3; let’s call it “Setup #1.” To start, prepare a data sheet as shown in Figure 5-15. Next, measure and fill in the “basic dimensions’’ at the top. Then, fill in the orientation direction, which is north in our example. Next, take a series of readings, zeroing at the top, and returning for final readings which should also be zero or nearly so. Now do a further check: Add the top and bottom readings algebraically (T + B), and add the side readings (S + S). The two sums should be equal, or nearly so. If the checks are poor, takc a new set of readings. Do the checks before accounting for bracket sag. Now, fill in the known or assumed bracket sag. If the bracket does not sag (optimist!), fill in zero. Combine the sag algebraically with the vertical rim reading as shown, and get the net reading using (+) or (-) as appropriate to accomplish the sag correction. A wellprepared form will have this sign printed on it. If it does not, mentally figure out what must be done to “un-sag” the bracket in the final position, and what sign would apply when doing so.
Now we are ready to interpret our data in the space provided on the form. To do this, first take half of our net rim reading:
Formullll
This is because we are looking for centerline rather than rim offset. Since its sign is minus, we can see from the indicator arrangement sketch that the machine element to be adjusted is higher than the stationary element, at the plane of measurement. This assumes the use of a conventional American dial indicator, in which a positive reading indicates contact point movement into the indicator.
By the same reasoning, we can see that the bottom face distance is .007 in. wider than the top face distance. Going now to the horizontal readings, we make the north rim reading zero by adding - .007 in. to it. To preserve the equality of our algebra,
we also add - .007 in. to the south rim reading, giving us - .029 in. Taking half of this, we find that the machine element to be adjusted is .0145 in. north of the stationary element at the plane of measurement. Finally, we do a similar operation on our horizontal face readings, and determine that the north face distance is wider by .014 in. The remaining part of the form provides space to put the calculated corrective
As you can see, the values found this way are close to those found earlier. The main problem people have with applying these formulas is choosing between plus and minus for the terms. The easiest way, in our opinion, is to visualize the “as found” conditions, and this will point the way that movement must proceed to go to zero misalignment. For example, our bottom face distance is wide-therefore we need to lower the feet (pivoting at plane A) which we denote with a minus sign. The machine element to be adjusted is higher at plane A-so we need to lower it some more, which takes another minus sign. For the horizontal, our north face distance is wider, so we need to move the feet north (again pivoting at plane A). The machine element to be adjusted is north at plane A, so we need to move it south. Call north plus or minus, so long as you call south the opposite sign. Not really hard, but a lot of people have trouble with the concept, which is why we prefer to concentrate on graphical methods , where direction of movement becomes more obvious. We will get into this shortly, but first let’s do a reverse-indicator problem mathematically.
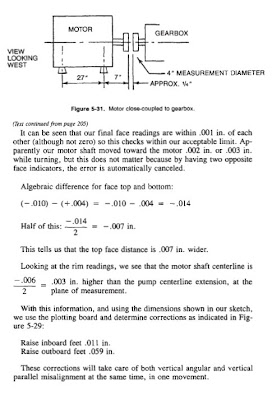
For our reverse-indicator example, we will use the setup shown earlier as Figure 5-6. Also, we must now refer to the appropriate data sheet, Figure 5-18. Finally, we resort to some triangles, Figures 5-19 and 5-20, to assist us in visualizing the situation.
Teknişk Resimmm
Graphical Techniques
Now let’s see how we can do the same thing more easily. To do this, we will turn to graphical techniques. Reference 7 shows one version of this in an article on alignment of barrel-type centrifugal compressors. Its author stretches a strip-chart out on a drafting table, and rules in the machine element shafts, to scale, oriented as shown by reverse-indicator readings, much as we did in our previous example. Movements to achieve zero offset, or to compensate for thermal growth, can then be plotted on the same sheet. This technique has the advantage of giving a complete and permanent written visual record of what is happening, and is especially useful on multi-element machinery
After reworking our first example with the alignment plotting board, we will proceed to our second example, using the reverse-indicator setup (setup 4). This is illustrated for us in Figures 5-18 and 5-24. We will then go on and give worked-out examples using the other setups in Figures 5-25 through 5-33.
Three-Indicator Face-and-Rim
Figure 5-27 depicts a pump with ball bearings driven by a motor having sleeve bearings. External obstructions prevent the use of setups 4 or 5, and the coupling surfaces have considerable runout, making setup 1 inadvisable. It is therefore decided to use setup 2-the three-indicator face-and-rim. A sag check shows 0.002 in. sag for the rim indicator bracket. Sag for the face indicators will be ignored. A preliminary straightedge alignment is made in the horizontal plane. Both shafts are then turned together and a set of vertical readings taken as shown on the accompanying data sheet in Figure 5-28.
Another graphical technique, which we prefer for most situations, is the Alignment Plotting Board. It is fast, easy, and accurate, particularly on the simple two-element trains comprising the majority of all machines. It can be used on multi-element trains, but is less efficient than graph paper beyond two elements. To our knowledge, the plotting board is the only method which handles both the face-and-rim and reverse-indicator setups with equal ease and can convert from R-I to F&R. An additional advantage is its portability-it is only 8V2 X 11 in. and is made from an “indestructible” polycarbonate plastic. Figure 5-22 shows it in use.
Let’s return to our first example. For convenience, we will refer to the data sheet in Figure 5-15, then show how to find the corrections on the plotting board. This is graphically explained in Figure 5-23. By flipping back and forth between data sheet and plotting board sketches, you can see where the answers come from. It will help to also read the instructions that come with the plotting board, if you have one. With practice, the answers will appear in a minute or two.
movements. Although these have been filled in for our example, let’s leave them for the time being, since we are not yet ready to explain the calculation procedure. We will show you how to get these numbers later. If you think you already know how, go ahead and try-the results may be interesting.
You have now seen the general idea about data recording and interpretation. By doing it systematically, on a prepared form corresponding to the actual field setup, you can minimize errors. If you are interrupted, you will not have to wonder what those numbers meant that you wrote down on the back of an envelope an hour ago. We will defer consideration of the remaining setups, until we have explained how to calculate alignment corrective movements. We will then take numerical examples for all the setups illustrated, and go through them all the way. Calculating the Corrective Movements
Many machinists make alignment corrective movements by trial and error. A conscientious person can easily spend two days aligning a machine this way, but by knowing how to calculate the corrections, the time can be cut to two hours or less.
Several methods, both manual and electronic, exist for doing such calculations. All, of course, are based on geometry, and some are rather complicated and difficult to follow. For those interested in such things, see Refercnces 1 through 15. Also, the alignment specialist should be aware of programmable calculator solutions. These make use of popular calculators such as the TI 59 and HP 67. By recording the alignment measurements on a prepared form, and entering these figures in the prescribed manner into the calculator, the required moves come out as answers. A variation of this is the TRS 80 pocket computer which has been programmed to do alignment calculations via successive instructions to the user telling him what information to enter.
By far the simplest calculator is the one described earlier in conjunction with the laser-based OPTALIGN@ system. Next to it, we rank the IMS calculator. l4 This is designed specifically “from the ground up’’ for the more common face-and-rim and reverse-indicator calculations, rather than being a standard commercial calculator or computer adapted for alignment. For the more complex multi-element trains, larger central computers can be used directly with telephone linkups. Ray Dodd’s book (Total Alignment, Reference 3) describes one such system. Another that we saw demonstrated provides not only the numerical data, but a diagrammatic representation of the machines and their alignment relationship, on a cathode ray tube.
The foregoing electronic systems are popular, and have advantages in speed, accuracy, and ease of use. They have disadvantages in cost, usability under adverse field and hazardous area conditions, pilferage, sensitivity to damage from temperature extremes and rough handling, and availability to the field machinist at 2:OO A.M. on a holiday weekend. They also, for the most part, work mainly with numbers, and the answers may require acceptance on blind faith. By contrast, graphical methods inherently aid visualization by showing the relationship of adjacent shaft centerlines to scale.
Manual calculation methods have the advantage of low investment (pencil and paper will suffice, but a sliderule or simple calculator will be faster). They have the disadvantage, some say, of requiring more thinking than the programmed electronic solutions, particularly to choose the plus and minus signs correctly.
The graphical methods, which we prefer, have the advantage of aiding visualization and avoiding confusion. Their accuracy will sometimes be lcss than that of the “pure” mathematical methods, but usually not enough to matter. Investment is low-graph paper and plotting boards are inexpensive. Speed is high once proficiency is attained, which usually does not take long.
In this text, we will emphasize the graphical approach. Before doing so, let’s highlight some common manual mathematical calculations. Nelson” published an explanation of one rather simple method a number of years ago. A shortened explanation is given in Figure 5-16. For our given example, this would work out as follows:
In any event, let us assume that we obtain readings of 0 and + 0.160 in. at the top and bottom vertical parallels respectively. We correct for sag in the following manner:
Bracket Sag Effect on Face Measurements
Bracket sag is generally thought to primarily affect rim readings, with little effect on face readings. Often this is true, but some risk may be incurred by assuming this without a test. Unlike rim sag, face sag effect depends not only on jig or bracket stiffness, but on its geometry. Determining face sag effect is fairly easy. First get rim sag for span to be used (we are referring here to the full indicator deflection due to sag when the setup is rotated from top to bottom). This may be obtained by trial, with rim indicator only, or from a graph of sags compiled for the bracket to be used. Then install a setup with rim indicator only, on Calibration pipe or on actual field machine, and “lay on” the face indicator and accessories, noting additional rim indicator deflection when this is done. Double this additional deflection, and add it to the rim sag found previously, if both the face and rim indicators are to be used simultaneously. If the face and the rim indicators are to be used separately, to reduce sag, use the original rim sag in the normal manner, and use this same original rim sag as shortly to be described in determining face sag effect-in this latter case utilizing a rim indicator installed temporarily with the face indicator for this purpose. If the face indicator is a different type (Le., different weight) from the rim indicator, obtain rim sag using this face indicator on the rim, and use this figure to determine face sag effect.
Now install face and rim setup on the actual machine, and zero the indicators. With indicators at the top, deflect bracket upward an amount equal to the appropriate rim sag, reading on the rim indicator, and note the face indicator reading. The face sag correction with indicators at bottom would be this amount with opposite sign. If zeroing the setup at the bottom, the face sag correction at the top would be this amount with same sign (if originally determined at top, as described).
Face Sag Effect-Examples
Example 1
Face and rim indicators are to be used together as shown in Figure 5-3. Assume you will obtain the following from your sag test: Rim sag with rim indicator only = .004 in. Rim sag with two indicators = .007 in. Mount the setup on the machine in the field, and with indicators at top, deflect the bracket upward .007 in. as measured on the rim indicator. When this is done, the face indicator reads plus .002 in. Face sag correction at the bottom position would therefore be minus .002 in. If you wish to zero at the bottom for alignment, but otherwise have data as noted, the face sag correction at the top would be plus .002 in.
Example 2
Face and rim indicators are to be used separately to reduce sag. Both indicators are the same type and weight. Other basic data are also the same.
Install face indicator and temporary rim indicator on the machine in the field, and place in top position. Zero indicators and deflect upward ,004 in. as measured on rim indicator. Face indicator reads plus .0013 in. Face sag correction at the bottom would therefore be minus .0013 in, If zeroing at the bottom for alignment, but otherwise the same as above, face sag correction at top would be plus .0013 in. Example 3
This will determine sag for “3-Indicator Face-and-Rim Setup” shown in Figure 5-4.
Set up the jig to the same geometry as for field installation but with rim indicator only and roll 180” top to bottom on pipe to get total single indicator rim sag - (Step 1).
Zero rim indicator on top and add or “lay on” face indicator, noting rim indicator deflection that occurs - (Step 2). Double this __ (Step 3).
Add it to original total single indicator rim sag (Step 1). - (Step 4). This figure, preceded by a plus sign, will be the sag correction for the rim indicator readings taken at bottom. With field measurement setup as shown, zero all indicators, and deflect the indicator end of the upper bracket upward an amount equal to the total rim sag (Step 4). Note the face sag effect by reading the face indicator. This amount, with opposite sign, is the face sag correction to apply to the readings taken at the lower position - (Step 5). Now deflect the upper bracket back down from its “total rim sag” deflection an amount equal to Step 3.
The amount of sag remaining on the face indicator, preceded by the same sign, is the sag correction for the single face indicator being read at the top position - (Step 6).
All of the foregoing refers of course to bracket sag. In long machines, we will also have shaft sag. This is mentioned only in passing, since there is no need to do anything about it at this time. Our “point-by-point” alignment will automatically take care of shaft sag. For initial leveling of large turbogenerators, etc., especially if using precision optical equipment, shaft sag must be considered. Manufacturers of such machines know this, and provide their erectors with suitable data for sag compensation. Further discussion of shaft sag is beyond the scope of this text.
Leveling Curved Surfaces
It is common practice to set up the “rim” dial indicators so their contact tips rest directly on the surface of coupling rims or shafts. If gross misalignment is not present, and if coupling and/or shaft diameters are large, which is usually the case, accuracy will often be adequate. If, however, major misalignment exists, and/or the rim or shaft diameters are small, a significant error is likely to be present. It occurs due to the measurement surface curvature, as illustrated in Figures 5-13 and 5-14. This error can usually be recognized by repeated failure of top-plusbottom (T + B) readings to equal side-plus-side (S + S) readings within one or two thousandths of an inch, and by calculated corrections resulting in an improvement which undershoots or overshoots and requires repeated corrections to achieve desired tolerance. A way to minimize this error is to use jigs, posts, and accessories which “square the circle.” Here we attach flat surfaces or posts to the curved surfaces, and level

them at top and bottom dead center. This corrects the error as shown in Figure 5-14.
For this method to be fully effective, rotation should be performed at accurate 90” quadrants, using inclinometer or bubble-vial device. In most cases, however, this error is not enough to bother eliminating- it is easier just to make a few more corrective moves, reducing the error each time.
Jig Posts
The preceding explanation showed a rudimentary auxiliary surface, or “jig post,” used for “squaring the circle.” A more common reason for using jig posts is to permit measurement without removing the spacer on a concealed hub gear coupling. If jig posts are used, it is important that they be used properly. In effect, we must ensure that the surfaces contacted by the indicators meet these criteria:
As already shown, they must be leveled in coordination at top and bottom dead centers, to avoid inclined plane error.
If any axial shaft movement can occur, as with sleeve bearings, the surfaces should also be made parallel to their shafts. This can be done by leveling axially at the top, rotating to the bottom, and rechecking. If bubble is not still level, tilt the surface back toward level for a half correction.
If face readings are to be taken on posts, the post face surfaces should be machined perpendicular to their rim surfaces. In addition to this, and to steps 1 and 2 just described, rotate shafts so posts are horizontal. Using a level, adjust face surfaces so they are vertical. Rotate 180” and recheck with level. If not still vertical, tilt back toward vertical to make a half correction on the bubble. This will accomplish our desired objective of getting the face surface perpendicular to the shaft in all measurement planes.
The foregoing assumes use of tri-axially adjustable jig posts. If such posts are not available, it may be possible to get good results using accurately machined nonadjustable posts. If readings and corrections do not turn out as desired, however, it could pay to make the level checks as described-they might pinpoint the problem and suggest a solution such as using a nonpost measurement setup.
interpretation and Data Recording
Due to sag as well as geometry of the machine installation, it is difficult and deceptive to try second-guessing the adequacy of alignment solely from the “raw” indicator readings. It is necessary to correct for sag, then note the “interpreted” readings, then plot or calculate these to see the overall picture-including equivalent face misalignment if primary readings were reverse-indicator on rims only. Sometimes thermal offsets must be included, which further complicates the overall picture. As a way to systematically consider these factors and arrive at a solution, it is helpful to use prepared data forms and stepwise calculation. Suppose we are using the two-indicator face-rim method shown in Figure 5-3; let’s call it “Setup #1.” To start, prepare a data sheet as shown in Figure 5-15. Next, measure and fill in the “basic dimensions’’ at the top. Then, fill in the orientation direction, which is north in our example. Next, take a series of readings, zeroing at the top, and returning for final readings which should also be zero or nearly so. Now do a further check: Add the top and bottom readings algebraically (T + B), and add the side readings (S + S). The two sums should be equal, or nearly so. If the checks are poor, takc a new set of readings. Do the checks before accounting for bracket sag. Now, fill in the known or assumed bracket sag. If the bracket does not sag (optimist!), fill in zero. Combine the sag algebraically with the vertical rim reading as shown, and get the net reading using (+) or (-) as appropriate to accomplish the sag correction. A wellprepared form will have this sign printed on it. If it does not, mentally figure out what must be done to “un-sag” the bracket in the final position, and what sign would apply when doing so.
Now we are ready to interpret our data in the space provided on the form. To do this, first take half of our net rim reading:
Formullll
This is because we are looking for centerline rather than rim offset. Since its sign is minus, we can see from the indicator arrangement sketch that the machine element to be adjusted is higher than the stationary element, at the plane of measurement. This assumes the use of a conventional American dial indicator, in which a positive reading indicates contact point movement into the indicator.
By the same reasoning, we can see that the bottom face distance is .007 in. wider than the top face distance. Going now to the horizontal readings, we make the north rim reading zero by adding - .007 in. to it. To preserve the equality of our algebra,
we also add - .007 in. to the south rim reading, giving us - .029 in. Taking half of this, we find that the machine element to be adjusted is .0145 in. north of the stationary element at the plane of measurement. Finally, we do a similar operation on our horizontal face readings, and determine that the north face distance is wider by .014 in. The remaining part of the form provides space to put the calculated corrective

Nelson’s method is easy to understand, and it works. It is basically a four-step procedure in this order:
1. Vertical angular Correction.
2. Vertical parallel correction.
3. Horizontal angular correction.
4. Horizontal parallel correction.
It has three disadvantages, however.
First, it requires four steps, whereas the more complex mathematical methods can combine angular and parallel data, resulting in a two-step correction. Secondly, it is quite likely that initial angular correction will subsequently have to be partially “un-done,” when making the corresponding parallel correction. Nobody likes to cut and install shims, then end up removing half of them. Finally, it is designed only for face-and-rim setups, and does not apply to the increasingly popular reverse-indicator technique.
We will now show two additional examples, wherein the angular and parallel correction are calculated at the same time, for an overall twostep correction. Frankly, we ourselves no longer use these methods, nor do we still use Nelson’s method, but are including them here for the sake of completeness. Graphical methods, as shown later, are easier and faster. In particular, the alignment plotting board should be judged extremely useful. Readers who are not interested in the mathematical method may wish to skip to page 192, where the much easier graphical methods are explained. But, in any event, here is the full mathematical treatment.
In our first example, we will reuse the data already given in our setup No. 1 data sheet.
First, we will solve for vertical corrections:
Using Nelson’s method, we found it necessary to make a 0.053 in. shim correction. Let us arbitrarily say this will be a shim addition beneath the inboard feet. At the coupling face, we then get a rise of:
1. Vertical angular Correction.
2. Vertical parallel correction.
3. Horizontal angular correction.
4. Horizontal parallel correction.
It has three disadvantages, however.
First, it requires four steps, whereas the more complex mathematical methods can combine angular and parallel data, resulting in a two-step correction. Secondly, it is quite likely that initial angular correction will subsequently have to be partially “un-done,” when making the corresponding parallel correction. Nobody likes to cut and install shims, then end up removing half of them. Finally, it is designed only for face-and-rim setups, and does not apply to the increasingly popular reverse-indicator technique.
We will now show two additional examples, wherein the angular and parallel correction are calculated at the same time, for an overall twostep correction. Frankly, we ourselves no longer use these methods, nor do we still use Nelson’s method, but are including them here for the sake of completeness. Graphical methods, as shown later, are easier and faster. In particular, the alignment plotting board should be judged extremely useful. Readers who are not interested in the mathematical method may wish to skip to page 192, where the much easier graphical methods are explained. But, in any event, here is the full mathematical treatment.
In our first example, we will reuse the data already given in our setup No. 1 data sheet.
First, we will solve for vertical corrections:
Using Nelson’s method, we found it necessary to make a 0.053 in. shim correction. Let us arbitrarily say this will be a shim addition beneath the inboard feet. At the coupling face, we then get a rise of:
As you can see, the values found this way are close to those found earlier. The main problem people have with applying these formulas is choosing between plus and minus for the terms. The easiest way, in our opinion, is to visualize the “as found” conditions, and this will point the way that movement must proceed to go to zero misalignment. For example, our bottom face distance is wide-therefore we need to lower the feet (pivoting at plane A) which we denote with a minus sign. The machine element to be adjusted is higher at plane A-so we need to lower it some more, which takes another minus sign. For the horizontal, our north face distance is wider, so we need to move the feet north (again pivoting at plane A). The machine element to be adjusted is north at plane A, so we need to move it south. Call north plus or minus, so long as you call south the opposite sign. Not really hard, but a lot of people have trouble with the concept, which is why we prefer to concentrate on graphical methods , where direction of movement becomes more obvious. We will get into this shortly, but first let’s do a reverse-indicator problem mathematically.
For our reverse-indicator example, we will use the setup shown earlier as Figure 5-6. Also, we must now refer to the appropriate data sheet, Figure 5-18. Finally, we resort to some triangles, Figures 5-19 and 5-20, to assist us in visualizing the situation.
Teknişk Resimmm
Graphical Techniques
Now let’s see how we can do the same thing more easily. To do this, we will turn to graphical techniques. Reference 7 shows one version of this in an article on alignment of barrel-type centrifugal compressors. Its author stretches a strip-chart out on a drafting table, and rules in the machine element shafts, to scale, oriented as shown by reverse-indicator readings, much as we did in our previous example. Movements to achieve zero offset, or to compensate for thermal growth, can then be plotted on the same sheet. This technique has the advantage of giving a complete and permanent written visual record of what is happening, and is especially useful on multi-element machinery
After reworking our first example with the alignment plotting board, we will proceed to our second example, using the reverse-indicator setup (setup 4). This is illustrated for us in Figures 5-18 and 5-24. We will then go on and give worked-out examples using the other setups in Figures 5-25 through 5-33.
Three-Indicator Face-and-Rim
Figure 5-27 depicts a pump with ball bearings driven by a motor having sleeve bearings. External obstructions prevent the use of setups 4 or 5, and the coupling surfaces have considerable runout, making setup 1 inadvisable. It is therefore decided to use setup 2-the three-indicator face-and-rim. A sag check shows 0.002 in. sag for the rim indicator bracket. Sag for the face indicators will be ignored. A preliminary straightedge alignment is made in the horizontal plane. Both shafts are then turned together and a set of vertical readings taken as shown on the accompanying data sheet in Figure 5-28.
trains such as the article discusses.
Another graphical technique, which we prefer for most situations, is the Alignment Plotting Board. It is fast, easy, and accurate, particularly on the simple two-element trains comprising the majority of all machines. It can be used on multi-element trains, but is less efficient than graph paper beyond two elements. To our knowledge, the plotting board is the only method which handles both the face-and-rim and reverse-indicator setups with equal ease and can convert from R-I to F&R. An additional advantage is its portability-it is only 8V2 X 11 in. and is made from an “indestructible” polycarbonate plastic. Figure 5-22 shows it in use.
Let’s return to our first example. For convenience, we will refer to the data sheet in Figure 5-15, then show how to find the corrections on the plotting board. This is graphically explained in Figure 5-23. By flipping back and forth between data sheet and plotting board sketches, you can see where the answers come from. It will help to also read the instructions that come with the plotting board, if you have one. With practice, the answers will appear in a minute or two.
movements. Although these have been filled in for our example, let’s leave them for the time being, since we are not yet ready to explain the calculation procedure. We will show you how to get these numbers later. If you think you already know how, go ahead and try-the results may be interesting.
You have now seen the general idea about data recording and interpretation. By doing it systematically, on a prepared form corresponding to the actual field setup, you can minimize errors. If you are interrupted, you will not have to wonder what those numbers meant that you wrote down on the back of an envelope an hour ago. We will defer consideration of the remaining setups, until we have explained how to calculate alignment corrective movements. We will then take numerical examples for all the setups illustrated, and go through them all the way. Calculating the Corrective Movements
Many machinists make alignment corrective movements by trial and error. A conscientious person can easily spend two days aligning a machine this way, but by knowing how to calculate the corrections, the time can be cut to two hours or less.
Several methods, both manual and electronic, exist for doing such calculations. All, of course, are based on geometry, and some are rather complicated and difficult to follow. For those interested in such things, see Refercnces 1 through 15. Also, the alignment specialist should be aware of programmable calculator solutions. These make use of popular calculators such as the TI 59 and HP 67. By recording the alignment measurements on a prepared form, and entering these figures in the prescribed manner into the calculator, the required moves come out as answers. A variation of this is the TRS 80 pocket computer which has been programmed to do alignment calculations via successive instructions to the user telling him what information to enter.
By far the simplest calculator is the one described earlier in conjunction with the laser-based OPTALIGN@ system. Next to it, we rank the IMS calculator. l4 This is designed specifically “from the ground up’’ for the more common face-and-rim and reverse-indicator calculations, rather than being a standard commercial calculator or computer adapted for alignment. For the more complex multi-element trains, larger central computers can be used directly with telephone linkups. Ray Dodd’s book (Total Alignment, Reference 3) describes one such system. Another that we saw demonstrated provides not only the numerical data, but a diagrammatic representation of the machines and their alignment relationship, on a cathode ray tube.
The foregoing electronic systems are popular, and have advantages in speed, accuracy, and ease of use. They have disadvantages in cost, usability under adverse field and hazardous area conditions, pilferage, sensitivity to damage from temperature extremes and rough handling, and availability to the field machinist at 2:OO A.M. on a holiday weekend. They also, for the most part, work mainly with numbers, and the answers may require acceptance on blind faith. By contrast, graphical methods inherently aid visualization by showing the relationship of adjacent shaft centerlines to scale.
Manual calculation methods have the advantage of low investment (pencil and paper will suffice, but a sliderule or simple calculator will be faster). They have the disadvantage, some say, of requiring more thinking than the programmed electronic solutions, particularly to choose the plus and minus signs correctly.
The graphical methods, which we prefer, have the advantage of aiding visualization and avoiding confusion. Their accuracy will sometimes be lcss than that of the “pure” mathematical methods, but usually not enough to matter. Investment is low-graph paper and plotting boards are inexpensive. Speed is high once proficiency is attained, which usually does not take long.
In this text, we will emphasize the graphical approach. Before doing so, let’s highlight some common manual mathematical calculations. Nelson” published an explanation of one rather simple method a number of years ago. A shortened explanation is given in Figure 5-16. For our given example, this would work out as follows:























Comments